Euler's Method

According to Heath in his book ``Scientific Computing'', 3rd edition. section 9.2 If we were to expand the Taylor expansion:

$$u(t+h) = u(t)+ u'(t) h + \frac{u''(t)}{2}h^2 + ...$$ (2.2)

where t represents a time step in function u.

Euler's method drops the terms of second order and higher to obtain the iterative approximation (in time steps):

$$u_{k+1} = u_{k}+ f(t_{k}, u_{k} ) h_{k}$$ (2.3)

we can easily see that $u' = f(t, u)$ and hence,

$$\frac{u_{k+1} - u_{k}}{h_{k}} = f(t_{k}, u_{k} )$$ (2.4)

is derived to show that the first derivative can be approximated this way. The second derivatives are derived in the repeated application of this method and hence get:

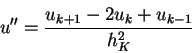$$u'' = \frac{u_{k+1}-2u_k+u_{k-1}}{h_K^2}$$ (2.5)

The intuition in Finite Difference method is taking the neighboring values into consideration to estimate the value in the center. Now say we have discretized an one-dimensional domain with regular interval into N points. Then for $2 \le i \le N-1$:

$$\frac{u^{k+1}_i - u^{k}_i}{s} = k \frac{u^k_{i+1} - 2u^k_{i} + u^k_{i-1} }{h^2}$$ (2.6)

We can easily expand that for two-dimensional domains which looks like this for $2 \le i \le N-1, 2 \le j \le N-1$

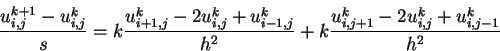$$\frac {u^{k+1}_{i,j} - u^{k}_{i,j}}{s} = k \frac{u^k_{i+1,... ...h^2} + k \frac{u^k_{i,j+1} - 2u^k_{i,j} + u^k_{i,j-1} } {h^2}$$ (2.7)

or

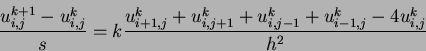$$\frac {u^{k+1}_{i,j} - u^{k}_{i,j}}{s} = k \frac{u^k_{i+1,... ... u^k_{i,j+1} + u^k_{i,j-1} + u^k_{i-1,j}- 4u^k_{i,j}} {h^2}$$ (2.8)